Balanced Binary Tree

1. Overview

In this tutorial, you will learn about a balanced binary tree and its different types. Also, you will find working examples of a balanced binary tree in C, C++, Java and Python.

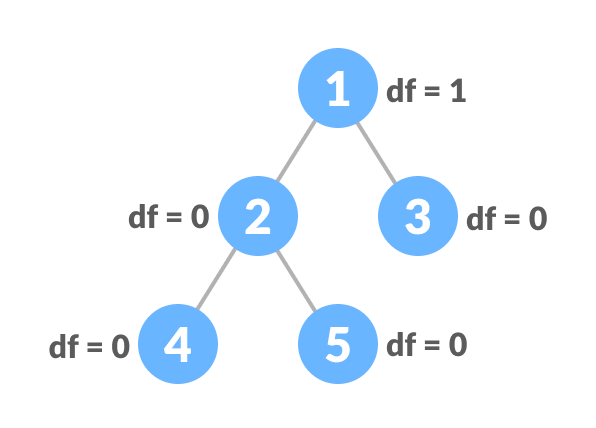
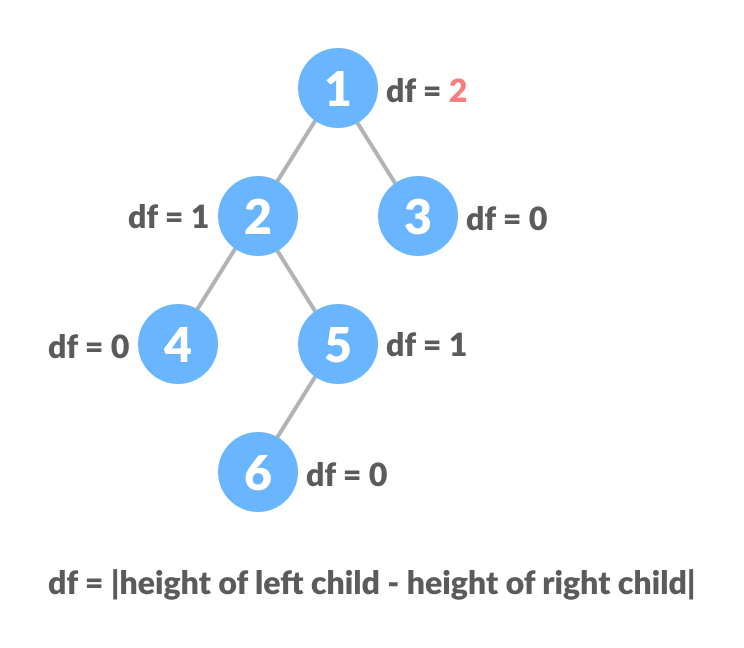
A balanced binary tree, also referred to as a height-balanced binary tree, is defined as a binary tree in which the height of the left and right subtree of any node differ by not more than 1.

To learn more about the height of a tree/node, visit Tree Data Structure.

Following are the conditions for a height-balanced binary tree:

1. difference between the left and the right subtree for any node is not more than one
2. the left subtree is balanced
3. the right subtree is balanced

2. Python, Java and C/C++ Examples

The following code is for checking whether a tree is height-balanced.

Source code by Python Language:

# Checking if a binary tree is height balanced in Python


class Node:

    def __init__(self, data):
        self.data = data
        self.left = self.right = None


class Height:
    def __init__(self):
        self.height = 0


def isHeightBalanced(root, height):

    left_height = Height()
    right_height = Height()

    if root is None:
        return True

    l = isHeightBalanced(root.left, left_height)
    r = isHeightBalanced(root.right, right_height)

    height.height = max(left_height.height, right_height.height) + 1

    if abs(left_height.height - right_height.height) 

Source code by Java Language:

// Checking if a binary tree is height balanced in Java

// Node creation
class Node {

  int data;
  Node left, right;

  Node(int d) {
    data = d;
    left = right = null;
  }
}

// Calculate height
class Height {
  int height = 0;
}

class BinaryTree {

  Node root;

  // Check height balance
  boolean checkHeightBalance(Node root, Height height) {

    // Check for emptiness
    if (root == null) {
      height.height = 0;
      return true;
    }

    Height leftHeighteight = new Height(), rightHeighteight = new Height();
    boolean l = checkHeightBalance(root.left, leftHeighteight);
    boolean r = checkHeightBalance(root.right, rightHeighteight);
    int leftHeight = leftHeighteight.height, rightHeight = rightHeighteight.height;

    height.height = (leftHeight > rightHeight ? leftHeight : rightHeight) + 1;

    if ((leftHeight - rightHeight >= 2) || (rightHeight - leftHeight >= 2))
      return false;

    else
      return l && r;
  }

  public static void main(String args[]) {
    Height height = new Height();

    BinaryTree tree = new BinaryTree();
    tree.root = new Node(1);
    tree.root.left = new Node(2);
    tree.root.right = new Node(3);
    tree.root.left.left = new Node(4);
    tree.root.left.right = new Node(5);

    if (tree.checkHeightBalance(tree.root, height))
      System.out.println("The tree is balanced");
    else
      System.out.println("The tree is not balanced");
  }
}

Source code by C Language:

// Checking if a binary tree is height balanced in C

#include <stdio.h>
#include <stdlib.h>
#define bool int

// Node creation
struct node {
  int item;
  struct node *left;
  struct node *right;
};

// Create a new node
struct node *newNode(int item) {
  struct node *node = (struct node *)malloc(sizeof(struct node));
  node->item = item;
  node->left = NULL;
  node->right = NULL;

  return (node);
}

// Check for height balance
bool checkHeightBalance(struct node *root, int *height) {
  // Check for emptiness
  int leftHeight = 0, rightHeight = 0;
  int l = 0, r = 0;

  if (root == NULL) {
    *height = 0;
    return 1;
  }

  l = checkHeightBalance(root->left, &leftHeight);
  r = checkHeightBalance(root->right, &rightHeight);

  *height = (leftHeight > rightHeight ? leftHeight : rightHeight) + 1;

  if ((leftHeight - rightHeight >= 2) || (rightHeight - leftHeight >= 2))
    return 0;

  else
    return l && r;
}

int main() {
  int height = 0;

  struct node *root = newNode(1);
  root->left = newNode(2);
  root->right = newNode(3);
  root->left->left = newNode(4);
  root->left->right = newNode(5);

  if (checkHeightBalance(root, &height))
    printf("The tree is balanced");
  else
    printf("The tree is not balanced");
}

Source code by C++ Language:

// Checking if a binary tree is height balanced in C++

#include 
using namespace std;

#define bool int

class node {
   public:
  int item;
  node *left;
  node *right;
};

// Create anew node
node *newNode(int item) {
  node *Node = new node();
  Node->item = item;
  Node->left = NULL;
  Node->right = NULL;

  return (Node);
}

// Check height balance
bool checkHeightBalance(node *root, int *height) {
  // Check for emptiness
  int leftHeight = 0, rightHeight = 0;

  int l = 0, r = 0;

  if (root == NULL) {
    *height = 0;
    return 1;
  }

  l = checkHeightBalance(root->left, &leftHeight);
  r = checkHeightBalance(root->right, &rightHeight);

  *height = (leftHeight > rightHeight ? leftHeight : rightHeight) + 1;

  if (std::abs(leftHeight - rightHeight >= 2))
    return 0;

  else
    return l && r;
}

int main() {
  int height = 0;

  node *root = newNode(1);
  root->left = newNode(2);
  root->right = newNode(3);
  root->left->left = newNode(4);
  root->left->right = newNode(5);

  if (checkHeightBalance(root, &height))
    cout << "The tree is balanced";
  else
    cout << "The tree is not balanced";
}

3. Balanced Binary Tree Applications

• AVL tree
• Balanced Binary Search Tree