Insertion into a B-tree - VietMX's Blog

Table of Contents

1. Insertion Operation
2. Insertion Example
3. Algorithm for Inserting an Element
4. Python, Java and C/C++ Examples

In this tutorial, you will learn how to insert a key into a btree. Also, you will find working examples of inserting keys into a B-tree in C, C++, Java and Python.

Inserting an element on a B-tree consists of two events: searching the appropriate node to insert the element and splitting the node if required.Insertion operation always takes place in the bottom-up approach.

Let us understand these events below.

1. Insertion Operation

If the tree is empty, allocate a root node and insert the key.
Update the allowed number of keys in the node.
Search the appropriate node for insertion.
If the node is full, follow the steps below.
Insert the elements in increasing order.
Now, there are elements greater than its limit. So, split at the median.
Push the median key upwards and make the left keys as a left child and the right keys as a right child.
If the node is not full, follow the steps below.
Insert the node in increasing order.

2. Insertion Example

Let us understand the insertion operation with the illustrations below.

The elements to be inserted are 8, 9, 10, 11, 15, 16, 17, 18, 20, 23.

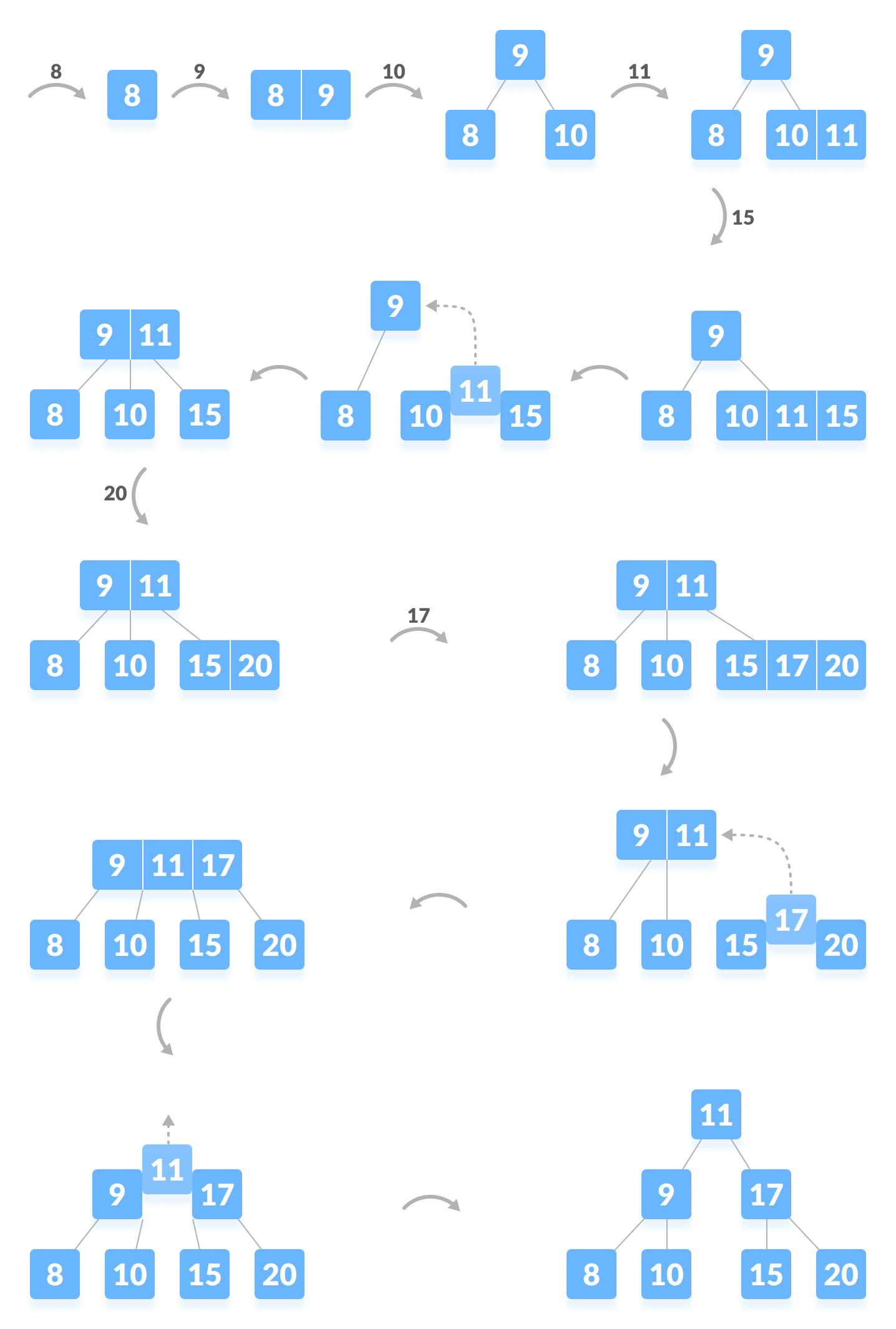
3. Algorithm for Inserting an Element

BreeInsertion(T, k)
r  root[T]
if n[r] = 2t - 1
    s = AllocateNode()
    root[T] = s
    leaf[s] = FALSE
    n[s] <- 0
    c1[s] <- r
    BtreeSplitChild(s, 1, r)
    BtreeInsertNonFull(s, k)
else BtreeInsertNonFull(r, k)
BtreeInsertNonFull(x, k)
i = n[x]
if leaf[x]
    while i ≥ 1 and k < keyi[x]
        keyi+1 [x] = keyi[x]
        i = i - 1
    keyi+1[x] = k
    n[x] = n[x] + 1
else while i ≥ 1 and k < keyi[x]
        i = i - 1
    i = i + 1
    if n[ci[x]] == 2t - 1
        BtreeSplitChild(x, i, ci[x])
        if k &rt; keyi[x]
            i = i + 1
    BtreeInsertNonFull(ci[x], k)
BtreeSplitChild(x, i)
BtreeSplitChild(x, i, y)
z = AllocateNode()
leaf[z] = leaf[y]
n[z] = t - 1
for j = 1 to t - 1
    keyj[z] = keyj+t[y]
if not leaf [y]
    for j = 1 to t
        cj[z] = cj + t[y]
n[y] = t - 1
for j = n[x] + 1 to i + 1
    cj+1[x] = cj[x]
ci+1[x] = z
for j = n[x] to i
    keyj+1[x] = keyj[x]
keyi[x] = keyt[y]
n[x] = n[x] + 1

4. Python, Java and C/C++ Examples

Source code by Python Language:

# Inserting a key on a B-tree in Python
 
 
# Create a node
class BTreeNode:
    def __init__(self, leaf=False):
        self.leaf = leaf
        self.keys = []
        self.child = []
 
 
# Tree
class BTree:
    def __init__(self, t):
        self.root = BTreeNode(True)
        self.t = t
 
    # Insert node
    def insert(self, k):
        root = self.root
        if len(root.keys) == (2 * self.t) - 1:
            temp = BTreeNode()
            self.root = temp
            temp.child.insert(0, root)
            self.split_child(temp, 0)
            self.insert_non_full(temp, k)
        else:
            self.insert_non_full(root, k)
 
    # Insert nonfull
    def insert_non_full(self, x, k):
        i = len(x.keys) - 1
        if x.leaf:
            x.keys.append((None, None))
            while i >= 0 and k[0] < x.keys[i][0]:
                x.keys[i + 1] = x.keys[i]
                i -= 1
            x.keys[i + 1] = k
        else:
            while i >= 0 and k[0] < x.keys[i][0]:
                i -= 1
            i += 1
            if len(x.child[i].keys) == (2 * self.t) - 1:
                self.split_child(x, i)
                if k[0] > x.keys[i][0]:
                    i += 1
            self.insert_non_full(x.child[i], k)
 
    # Split the child
    def split_child(self, x, i):
        t = self.t
        y = x.child[i]
        z = BTreeNode(y.leaf)
        x.child.insert(i + 1, z)
        x.keys.insert(i, y.keys[t - 1])
        z.keys = y.keys[t: (2 * t) - 1]
        y.keys = y.keys[0: t - 1]
        if not y.leaf:
            z.child = y.child[t: 2 * t]
            y.child = y.child[0: t - 1]
 
    # Print the tree
    def print_tree(self, x, l=0):
        print("Level ", l, " ", len(x.keys), end=":")
        for i in x.keys:
            print(i, end=" ")
        print()
        l += 1
        if len(x.child) > 0:
            for i in x.child:
                self.print_tree(i, l)
 
 
def main():
    B = BTree(3)
 
    for i in range(10):
        B.insert((i, 2 * i))
 
    B.print_tree(B.root)
 
 
if __name__ == '__main__':
    main()

Source code by Java Language:

// Inserting a key on a B-tree in Java 
 
public class BTree {
 
  private int T;
 
  // Node Creation
  public class Node {
    int n;
    int key[] = new int[2 * T - 1];
    Node child[] = new Node[2 * T];
    boolean leaf = true;
 
    public int Find(int k) {
      for (int i = 0; i < this.n; i++) {
        if (this.key[i] == k) {
          return i;
        }
      }
      return -1;
    };
  }
 
  public BTree(int t) {
    T = t;
    root = new Node();
    root.n = 0;
    root.leaf = true;
  }
 
  private Node root;
 
  // split
  private void split(Node x, int pos, Node y) {
    Node z = new Node();
    z.leaf = y.leaf;
    z.n = T - 1;
    for (int j = 0; j < T - 1; j++) {
      z.key[j] = y.key[j + T];
    }
    if (!y.leaf) {
      for (int j = 0; j < T; j++) {
        z.child[j] = y.child[j + T];
      }
    }
    y.n = T - 1;
    for (int j = x.n; j >= pos + 1; j--) {
      x.child[j + 1] = x.child[j];
    }
    x.child[pos + 1] = z;
 
    for (int j = x.n - 1; j >= pos; j--) {
      x.key[j + 1] = x.key[j];
    }
    x.key[pos] = y.key[T - 1];
    x.n = x.n + 1;
  }
 
  // insert key
  public void insert(final int key) {
    Node r = root;
    if (r.n == 2 * T - 1) {
      Node s = new Node();
      root = s;
      s.leaf = false;
      s.n = 0;
      s.child[0] = r;
      split(s, 0, r);
      _insert(s, key);
    } else {
      _insert(r, key);
    }
  }
 
  // insert node
  final private void _insert(Node x, int k) {
 
    if (x.leaf) {
      int i = 0;
      for (i = x.n - 1; i >= 0 && k < x.key[i]; i--) {
        x.key[i + 1] = x.key[i];
      }
      x.key[i + 1] = k;
      x.n = x.n + 1;
    } else {
      int i = 0;
      for (i = x.n - 1; i >= 0 && k < x.key[i]; i--) {
      }
      ;
      i++;
      Node tmp = x.child[i];
      if (tmp.n == 2 * T - 1) {
        split(x, i, tmp);
        if (k > x.key[i]) {
          i++;
        }
      }
      _insert(x.child[i], k);
    }
 
  }
 
  public void display() {
    display(root);
  }
 
  // Display the tree
  private void display(Node x) {
    assert (x == null);
    for (int i = 0; i < x.n; i++) {
      System.out.print(x.key[i] + " ");
    }
    if (!x.leaf) {
      for (int i = 0; i < x.n + 1; i++) {
        display(x.child[i]);
      }
    }
  }
 
  public static void main(String[] args) {
    BTree b = new BTree(3);
    b.insert(8);
    b.insert(9);
    b.insert(10);
    b.insert(11);
    b.insert(15);
    b.insert(20);
    b.insert(17);
 
    b.display();
  }
}

Source code by C Language:

// insertioning a key on a B-tree in C
 
#include <stdio.h>
#include <stdlib.h>
 
#define MAX 3
#define MIN 2
 
struct btreeNode {
  int item[MAX + 1], count;
  struct btreeNode *link[MAX + 1];
};
 
struct btreeNode *root;
 
// Node creation
struct btreeNode *createNode(int item, struct btreeNode *child) {
  struct btreeNode *newNode;
  newNode = (struct btreeNode *)malloc(sizeof(struct btreeNode));
  newNode->item[1] = item;
  newNode->count = 1;
  newNode->link[0] = root;
  newNode->link[1] = child;
  return newNode;
}
 
// Insert
void insertValue(int item, int pos, struct btreeNode *node,
          struct btreeNode *child) {
  int j = node->count;
  while (j > pos) {
    node->item[j + 1] = node->item[j];
    node->link[j + 1] = node->link[j];
    j--;
  }
  node->item[j + 1] = item;
  node->link[j + 1] = child;
  node->count++;
}
 
// Split node
void splitNode(int item, int *pval, int pos, struct btreeNode *node,
         struct btreeNode *child, struct btreeNode **newNode) {
  int median, j;
 
  if (pos > MIN)
    median = MIN + 1;
  else
    median = MIN;
 
  *newNode = (struct btreeNode *)malloc(sizeof(struct btreeNode));
  j = median + 1;
  while (j <= MAX) {
    (*newNode)->item[j - median] = node->item[j];
    (*newNode)->link[j - median] = node->link[j];
    j++;
  }
  node->count = median;
  (*newNode)->count = MAX - median;
 
  if (pos <= MIN) {
    insertValue(item, pos, node, child);
  } else {
    insertValue(item, pos - median, *newNode, child);
  }
  *pval = node->item[node->count];
  (*newNode)->link[0] = node->link[node->count];
  node->count--;
}
 
// Set the value of node
int setNodeValue(int item, int *pval,
           struct btreeNode *node, struct btreeNode **child) {
  int pos;
  if (!node) {
    *pval = item;
    *child = NULL;
    return 1;
  }
 
  if (item < node->item[1]) {
    pos = 0;
  } else {
    for (pos = node->count;
       (item < node->item[pos] && pos > 1); pos--)
      ;
    if (item == node->item[pos]) {
      printf("Duplicates not allowed\n");
      return 0;
    }
  }
  if (setNodeValue(item, pval, node->link[pos], child)) {
    if (node->count < MAX) {
      insertValue(*pval, pos, node, *child);
    } else {
      splitNode(*pval, pval, pos, node, *child, child);
      return 1;
    }
  }
  return 0;
}
 
// Insert the value
void insertion(int item) {
  int flag, i;
  struct btreeNode *child;
 
  flag = setNodeValue(item, &i, root, &child);
  if (flag)
    root = createNode(i, child);
}
 
// Copy the successor
void copySuccessor(struct btreeNode *myNode, int pos) {
  struct btreeNode *dummy;
  dummy = myNode->link[pos];
 
  for (; dummy->link[0] != NULL;)
    dummy = dummy->link[0];
  myNode->item[pos] = dummy->item[1];
}
 
// Do rightshift
void rightShift(struct btreeNode *myNode, int pos) {
  struct btreeNode *x = myNode->link[pos];
  int j = x->count;
 
  while (j > 0) {
    x->item[j + 1] = x->item[j];
    x->link[j + 1] = x->link[j];
  }
  x->item[1] = myNode->item[pos];
  x->link[1] = x->link[0];
  x->count++;
 
  x = myNode->link[pos - 1];
  myNode->item[pos] = x->item[x->count];
  myNode->link[pos] = x->link[x->count];
  x->count--;
  return;
}
 
// Do leftshift
void leftShift(struct btreeNode *myNode, int pos) {
  int j = 1;
  struct btreeNode *x = myNode->link[pos - 1];
 
  x->count++;
  x->item[x->count] = myNode->item[pos];
  x->link[x->count] = myNode->link[pos]->link[0];
 
  x = myNode->link[pos];
  myNode->item[pos] = x->item[1];
  x->link[0] = x->link[1];
  x->count--;
 
  while (j <= x->count) {
    x->item[j] = x->item[j + 1];
    x->link[j] = x->link[j + 1];
    j++;
  }
  return;
}
 
// Merge the nodes
void mergeNodes(struct btreeNode *myNode, int pos) {
  int j = 1;
  struct btreeNode *x1 = myNode->link[pos], *x2 = myNode->link[pos - 1];
 
  x2->count++;
  x2->item[x2->count] = myNode->item[pos];
  x2->link[x2->count] = myNode->link[0];
 
  while (j <= x1->count) {
    x2->count++;
    x2->item[x2->count] = x1->item[j];
    x2->link[x2->count] = x1->link[j];
    j++;
  }
 
  j = pos;
  while (j < myNode->count) {
    myNode->item[j] = myNode->item[j + 1];
    myNode->link[j] = myNode->link[j + 1];
    j++;
  }
  myNode->count--;
  free(x1);
}
 
// Adjust the node
void adjustNode(struct btreeNode *myNode, int pos) {
  if (!pos) {
    if (myNode->link[1]->count > MIN) {
      leftShift(myNode, 1);
    } else {
      mergeNodes(myNode, 1);
    }
  } else {
    if (myNode->count != pos) {
      if (myNode->link[pos - 1]->count > MIN) {
        rightShift(myNode, pos);
      } else {
        if (myNode->link[pos + 1]->count > MIN) {
          leftShift(myNode, pos + 1);
        } else {
          mergeNodes(myNode, pos);
        }
      }
    } else {
      if (myNode->link[pos - 1]->count > MIN)
        rightShift(myNode, pos);
      else
        mergeNodes(myNode, pos);
    }
  }
}
 
// Traverse the tree
void traversal(struct btreeNode *myNode) {
  int i;
  if (myNode) {
    for (i = 0; i < myNode->count; i++) {
      traversal(myNode->link[i]);
      printf("%d ", myNode->item[i + 1]);
    }
    traversal(myNode->link[i]);
  }
}
 
int main() {
  int item, ch;
 
  insertion(8);
  insertion(9);
  insertion(10);
  insertion(11);
  insertion(15);
  insertion(16);
  insertion(17);
  insertion(18);
  insertion(20);
  insertion(23);
 
  traversal(root);
}

Source code by C++ Language:

// Inserting a key on a B-tree in C++
 
#include <iostream>
using namespace std;
 
class Node {
  int *keys;
  int t;
  Node **C;
  int n;
  bool leaf;
 
   public:
  Node(int _t, bool _leaf);
 
  void insertNonFull(int k);
  void splitChild(int i, Node *y);
  void traverse();
 
  friend class BTree;
};
 
class BTree {
  Node *root;
  int t;
 
   public:
  BTree(int _t) {
    root = NULL;
    t = _t;
  }
 
  void traverse() {
    if (root != NULL)
      root->traverse();
  }
 
  void insert(int k);
};
 
Node::Node(int t1, bool leaf1) {
  t = t1;
  leaf = leaf1;
 
  keys = new int[2 * t - 1];
  C = new Node *[2 * t];
 
  n = 0;
}
 
// Traverse the nodes
void Node::traverse() {
  int i;
  for (i = 0; i < n; i++) {
    if (leaf == false)
      C[i]->traverse();
    cout << " " << keys[i];
  }
 
  if (leaf == false)
    C[i]->traverse();
}
 
// Insert the node
void BTree::insert(int k) {
  if (root == NULL) {
    root = new Node(t, true);
    root->keys[0] = k;
    root->n = 1;
  } else {
    if (root->n == 2 * t - 1) {
      Node *s = new Node(t, false);
 
      s->C[0] = root;
 
      s->splitChild(0, root);
 
      int i = 0;
      if (s->keys[0] < k)
        i++;
      s->C[i]->insertNonFull(k);
 
      root = s;
    } else
      root->insertNonFull(k);
  }
}
 
// Insert non full condition
void Node::insertNonFull(int k) {
  int i = n - 1;
 
  if (leaf == true) {
    while (i >= 0 && keys[i] > k) {
      keys[i + 1] = keys[i];
      i--;
    }
 
    keys[i + 1] = k;
    n = n + 1;
  } else {
    while (i >= 0 && keys[i] > k)
      i--;
 
    if (C[i + 1]->n == 2 * t - 1) {
      splitChild(i + 1, C[i + 1]);
 
      if (keys[i + 1] < k)
        i++;
    }
    C[i + 1]->insertNonFull(k);
  }
}
 
// split the child
void Node::splitChild(int i, Node *y) {
  Node *z = new Node(y->t, y->leaf);
  z->n = t - 1;
 
  for (int j = 0; j < t - 1; j++)
    z->keys[j] = y->keys[j + t];
 
  if (y->leaf == false) {
    for (int j = 0; j < t; j++)
      z->C[j] = y->C[j + t];
  }
 
  y->n = t - 1;
  for (int j = n; j >= i + 1; j--)
    C[j + 1] = C[j];
 
  C[i + 1] = z;
 
  for (int j = n - 1; j >= i; j--)
    keys[j + 1] = keys[j];
 
  keys[i] = y->keys[t - 1];
  n = n + 1;
}
 
int main() {
  BTree t(3);
  t.insert(8);
  t.insert(9);
  t.insert(10);
  t.insert(11);
  t.insert(15);
  t.insert(16);
  t.insert(17);
  t.insert(18);
  t.insert(20);
  t.insert(23);
 
  cout << "The B-tree is: ";
  t.traverse();
}