Perfect Binary Tree

1. Overview

In this tutorial, you will learn about the perfect binary tree. Also, you will find working examples for checking a perfect binary tree in C, C++, Java and Python.

A perfect binary tree is a type of binary tree in which every internal node has exactly two child nodes and all the leaf nodes are at the same level.

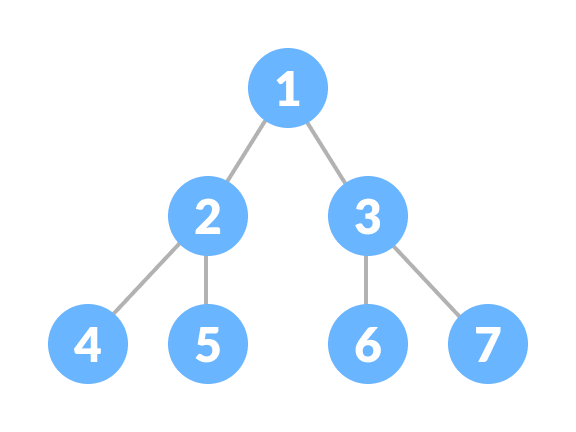
All the internal nodes have a degree of 2.

Recursively, a perfect binary tree can be defined as:

1. If a single node has no children, it is a perfect binary tree of height h = 0,
2. If a node has h > 0, it is a perfect binary tree if both of its subtrees are of height h - 1 and are non-overlapping.

2. Python, Java and C/C++ Examples

The following code is for checking whether a tree is a perfect binary tree.

Source code by Python Language:

# Checking if a binary tree is a perfect binary tree in Python


class newNode:
    def __init__(self, k):
        self.key = k
        self.right = self.left = None


# Calculate the depth
def calculateDepth(node):
    d = 0
    while (node is not None):
        d += 1
        node = node.left
    return d


# Check if the tree is perfect binary tree
def is_perfect(root, d, level=0):

    # Check if the tree is empty
    if (root is None):
        return True

    # Check the presence of trees
    if (root.left is None and root.right is None):
        return (d == level + 1)

    if (root.left is None or root.right is None):
        return False

    return (is_perfect(root.left, d, level + 1) and
            is_perfect(root.right, d, level + 1))


root = None
root = newNode(1)
root.left = newNode(2)
root.right = newNode(3)
root.left.left = newNode(4)
root.left.right = newNode(5)

if (is_perfect(root, calculateDepth(root))):
    print("The tree is a perfect binary tree")
else:
    print("The tree is not a perfect binary tree")

Source code by Java Language:

// Checking if a binary tree is a perfect binary tree in Java

class PerfectBinaryTree {

  static class Node {
    int key;
    Node left, right;
  }

  // Calculate the depth
  static int depth(Node node) {
    int d = 0;
    while (node != null) {
      d++;
      node = node.left;
    }
    return d;
  }

  // Check if the tree is perfect binary tree
  static boolean is_perfect(Node root, int d, int level) {

    // Check if the tree is empty
    if (root == null)
      return true;

    // If for children
    if (root.left == null && root.right == null)
      return (d == level + 1);

    if (root.left == null || root.right == null)
      return false;

    return is_perfect(root.left, d, level + 1) && is_perfect(root.right, d, level + 1);
  }

  // Wrapper function
  static boolean is_Perfect(Node root) {
    int d = depth(root);
    return is_perfect(root, d, 0);
  }

  // Create a new node
  static Node newNode(int k) {
    Node node = new Node();
    node.key = k;
    node.right = null;
    node.left = null;
    return node;
  }

  public static void main(String args[]) {
    Node root = null;
    root = newNode(1);
    root.left = newNode(2);
    root.right = newNode(3);
    root.left.left = newNode(4);
    root.left.right = newNode(5);

    if (is_Perfect(root) == true)
      System.out.println("The tree is a perfect binary tree");
    else
      System.out.println("The tree is not a perfect binary tree");
  }
}

Source code by C Language:

// Checking if a binary tree is a perfect binary tree in C

#include <stdbool.h>
#include <stdio.h>
#include <stdlib.h>

struct node {
  int data;
  struct node *left;
  struct node *right;
};

// Creating a new node
struct node *newnode(int data) {
  struct node *node = (struct node *)malloc(sizeof(struct node));
  node->data = data;
  node->left = NULL;
  node->right = NULL;

  return (node);
}

// Calculate the depth
int depth(struct node *node) {
  int d = 0;
  while (node != NULL) {
    d++;
    node = node->left;
  }
  return d;
}

// Check if the tree is perfect
bool is_perfect(struct node *root, int d, int level) {
    // Check if the tree is empty
  if (root == NULL)
    return true;

  // Check the presence of children
  if (root->left == NULL && root->right == NULL)
    return (d == level + 1);

  if (root->left == NULL || root->right == NULL)
    return false;

  return is_perfect(root->left, d, level + 1) &&
       is_perfect(root->right, d, level + 1);
}

// Wrapper function
bool is_Perfect(struct node *root) {
  int d = depth(root);
  return is_perfect(root, d, 0);
}

int main() {
  struct node *root = NULL;
  root = newnode(1);
  root->left = newnode(2);
  root->right = newnode(3);
  root->left->left = newnode(4);
  root->left->right = newnode(5);
  root->right->left = newnode(6);

  if (is_Perfect(root))
    printf("The tree is a perfect binary tree\n");
  else
    printf("The tree is not a perfect binary tree\n");
}

Source code by C++ Language:

// Checking if a binary tree is a perfect binary tree in C++

#include <iostream>
using namespace std;

struct Node {
  int key;
  struct Node *left, *right;
};

int depth(Node *node) {
  int d = 0;
  while (node != NULL) {
    d++;
    node = node->left;
  }
  return d;
}

bool isPerfectR(struct Node *root, int d, int level = 0) {
  if (root == NULL)
    return true;

  if (root->left == NULL && root->right == NULL)
    return (d == level + 1);

  if (root->left == NULL || root->right == NULL)
    return false;

  return isPerfectR(root->left, d, level + 1) &&
       isPerfectR(root->right, d, level + 1);
}

bool isPerfect(Node *root) {
  int d = depth(root);
  return isPerfectR(root, d);
}

struct Node *newNode(int k) {
  struct Node *node = new Node;
  node->key = k;
  node->right = node->left = NULL;
  return node;
}

int main() {
  struct Node *root = NULL;
  root = newNode(1);
  root->left = newNode(2);
  root->right = newNode(3);
  root->left->left = newNode(4);
  root->left->right = newNode(5);
  root->right->left = newNode(6);

  if (isPerfect(root))
    cout << "The tree is a perfect binary tree\n";
  else
    cout << "The tree is not a perfect binary tree\n";
}

3. Perfect Binary Tree Theorems

1. A perfect binary tree of height h has 2h + 1 – 1 node.
2. A perfect binary tree with n nodes has height log(n + 1) – 1 = Θ(ln(n)).
3. A perfect binary tree of height h has 2h leaf nodes.
4. The average depth of a node in a perfect binary tree is Θ(ln(n)).